Chemistry 30

3.1 Solubility Curves

There are charts and tables available that we can use to get an idea of how soluble a certain solute is in a certain solvent. We will take a look at two of them in these next two sections.

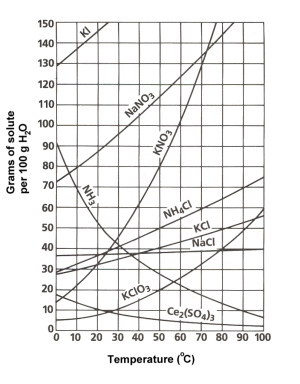
Solubility curves, like the one shown here, tell us what mass of solute will dissolve in 100g (or 100mL; see note) of water over a range of temperatures.

You'll want to have a copy of this figure handy - click on the graph to get a larger version, then print out the graph (or click for a pdf version).

You'll notice that for most substances, solubility increases as temperature increases. As discussed earlier (Section 1.3), in solutions involving liquids and solids typically more solute can be dissolved at higher temperatures. Can you find any exceptions on the chart?

Here's an example of reading the chart. Find the curve for KClO₃.

At 30°C approximately 10g of KClO₃ will dissolve in 100g of water. If the temperature is increased to 80°C, approximately 40g of the substance will dissolve in 100g (or 100mL) of water.

Here are some for you to try.

What mass of solute will dissolve in 100mL of water at the following temperatures. Also determine which of the three substances is most soluble in water at 15°C.

1.	KNO3at 70°C
2.	NaCl at 100°C
3.	NH4Cl at 90°C

Solution:

		Solubility
1.	KNO3at 70°C	140g / 100mL
2.	NaCl at 100°C	40g / 100mL
3.	NH4Cl at 90°C	72g / 100mL
4.	NaCl is the most soluble at 15°C

---

On a solubility curve, the lines indicate the concentration of a saturated solution - the maximum amount of solute that will dissolve at that specific temperature. The molar concentration of the substance can be calculated, as shown by this example:

Determine the molarity of a saturated NaCl solution at 25°C.

Solution

We can see from the curve that about 38 g of NaCl dissolves in 100mL at 25°C. Molarity, M, has the units mol·L^-1, so we want to convert 38g ·100mL^-1 to mol·L^-1. We will use unit analysis to do this. First, however, let's convert mL to L:

38g 100mL

×

10 10

=

380g 1,000mL

=

380g 1L

Since we need to convert from grams to moles, we will need to use the molar mass of NaCl, which is 58.5 g·mol^-1

desired unit				molar mass		answer
mol L	=	380g L	×	1 mol 58.5g	=	6.5 mol L	OR	6.5M
								answer

---

Values on the graph below a curve represent unsaturated solutions - more solute could be dissolved at that temperature. Values above a curve represent supersaturated solutions, a solution which holds more solute that can normally dissolve in that volume of solvent.

Some examples:

What term - saturated, unsaturated, or supersaturated - best describes:

• a solution that contains 70g of NaNO₃ per 100 mL H₂O at 30°C
• a solution that contains 60g of dissolved KCl per 100 mL H₂O at 80°C

Solution

• The NaNO₃ solution is unsaturated. At 30°C a saturated solution would be able to dissolve approximately 95 g of NaNO₃ . Since there are only 70g in the solution, 25 more grams of NaNO₃ could be added and it would all dissolve.
  
• The KCl solution is supersaturated. At 80°C a saturated KCl solution contains 50 g KCl per 100 mL H₂O. This solution is holding 10 g of excess KCl.

If a small crystal of KCl is added to this supersaturated solution, the excess KCl will immediately come out of solution. Have a look!

---

Lab Activity The Solubility of a Salt

The next section examines solubility tables.

 

---

NOTE: Since the density of water is 1.0 g·mL^-1, 1.0 gram of water will have a volume of 1.0 mL and vice versa.

return to notes